Tuned Mass Damper System

L

1

1.

L

2

0.6

m

1

0.2

m

2

0.8

k

1

0.6

k

2

0.4

b

0.

x

0,1

0.25

x

0,2

0.5

p

0,1

0.

p

0,2

0.

t

100.

plot

{

x

1

(t),

p

1

(t),

p

2

(t)}

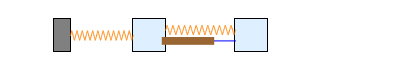
Tuned mass damper systems have been developed in recent years to diminish the oscillations of buildings during earthquakes. A large mass is hung by springs at the top of the building. The oscillations of the mass dissipates the energy into heat by means of a damper.

This Demonstration shows a system with two masses,

m

1

and

m

2

. Let

x

1

and

x

2

be the positions of the masses,

L

1

and

L

2

be the lengths of the springs, and

k

1

and

k

2

be the spring constants. The effect of the damper can be represented by a damping coefficient

b

in the system. The energy decreases whenever the second mass is moving relative to the first. It is said that the damper is "tuned" when the optimal value of

k

2

is selected for a specific building. Hamilton's equations of motion are solved for the initial conditions

x

0,i

and

p

0,i

(positions and momenta). Their plots are shown for all three possible combinations of three variables. Below the plot, the state of the masses with the springs and the damper is shown as a function of time.