Threefold Symmetry from Rotated Plane Waves

function

z

g

1

g

2

g

3

f

3

f

4

f

5

f

6

f

7

plot range

0.5

1

1.5

2

plot points factor

1

4

9

16

25

36

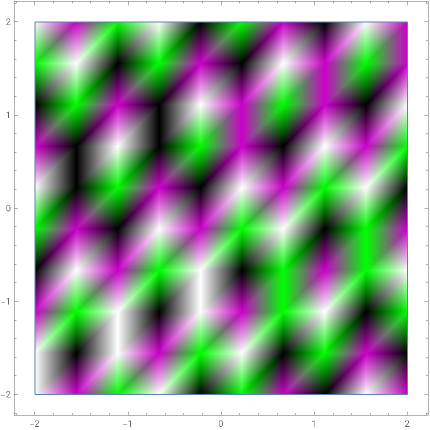
The plot of the function

g

0

(z)=f(z)=

2πiIm(z)

e

represents a plane wave periodic in the direction of the imaginary axis. Threefold symmetry is created by taking the mean of the functions

g

0

(z)

,

g

1

(z)=f(

ω

3

z)=

πi-Im(z)+

3

Re(z)

e

, and

g

2

(z)=f

2

ω

3

z=

πi-Im(z)-

3

Re(z)

e

, where

ω

3

=(-1±i

3

)2

are the two complex roots of the equation

3

z

=1

. So

f

3

(z)=

1

3

(

g

1

(z)+

g

2

(z)+

g

3

(z))

is invariant under rotation by

2π/3

; in other words, it has threefold symmetry.

Similarly,

f

n

(z)

has

n

-fold symmetry for

n=4,5,6,7

, using the

th

n

roots of unity, the solutions of

n

z

=1

.