Tarski's Adaptation of Wojtowicz's Argument on Optimal Dissection of a Unit Square

AB

3.3

show dissection

move

0

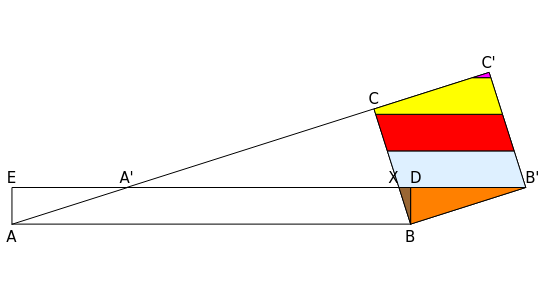
This Demonstration shows a reconstruction of a theorem of Tarski. An optimal dissection uses the smallest number of pieces. The theorem states that the number of pieces in an optimal dissection of a unit square into a rectangle of dimensions

x>1

and

1/x

has an upper bound

2+

2

x

-1



, where

⌈x⌉

denotes the ceiling of

x

, that is, the smallest integer greater than or equal to

x

.

In the case

x=3.5

, this gives a six-piece dissection.