Tangents to the Circumcircle at the Vertices

s(A)

≈

9.26

s(B)

≈

0.02

s(C)

≈

1.40

s(C'B')

≈

9.26

s(C'A')

≈

0.02

s(A'B')

≈

1.40

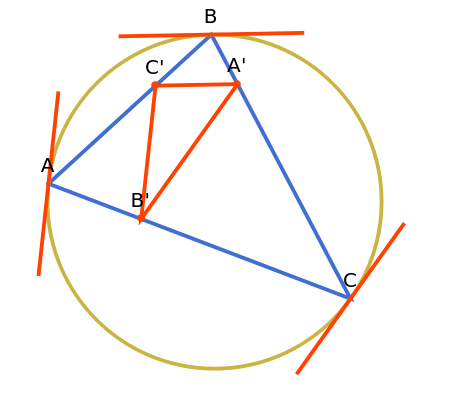
Let ABC be a triangle and A'B'C' its orthic triangle. The tangent lines to the circumcircle of ABC at the vertices are parallel to the corresponding sides of the orthic triangle A'B'C'. For example, the tangent line at A is parallel to B'C'.

In the table,

s(X)

is the slope of the tangent line to the circumcircle at the point X and

s(XY)

is the slope of the line segment XY.