Stability and Critical Angle of a Box

center of gravity

h

1

base length

t

2

tipping angle

θ

0

test for balance

test

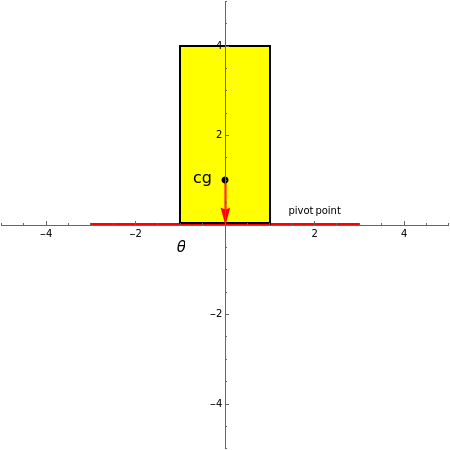
If you tilt a box, one edge of the base of support becomes a pivot point. As long as the center of gravity of the box remains over the base of support, torque due to gravity rotates the object back toward its stable equilibrium position; we say that the object is stable. But if the center of gravity moves outside the base of support, the gravitational torque causes a rotation in the opposite direction. Now the box rolls over; it is unstable. A critical angle

θ

c

is reached when the center of gravity is directly over the pivot point. This is the point of balance, with no net torque. For vehicles, the distance between the tires—the base of support—is called the track width

t

. If the height of the center of gravity is

h

, you can see from the Demonstration that the critical angle is

θ

c

=

-1

tan

t/2

h

=

-1

tan

t

2h

.