Prüfer Encoding of Labeled Trees

number of vertices

5

6

7

8

9

10

11

12

13

14

15

random tree

{3,3,3}

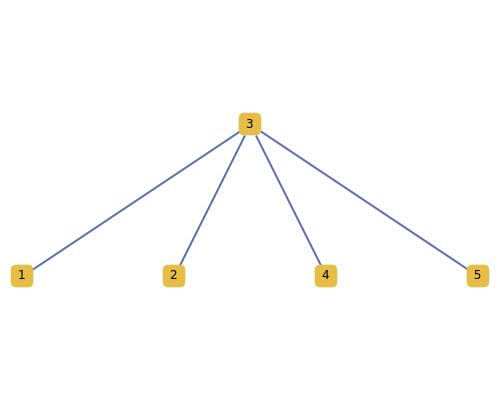
A Prüfer sequence of length

n-2

, for

n>1

, is any sequence of integers between 1 and

n

with repetitions allowed. There is a one-to-one correspondence between the set of labeled trees with

n

vertices and the Prüfer sequences of length

n-2

, from which is derived Cayley's formula that counts the number of labeled trees of

n

vertices, namely

n-2

n

. This Demonstration shows the Prüfer sequence of random labeled trees of a chosen number of vertices. The procedure is as follows. Choose a leaf (a vertex of degree 1) with the smallest label and write down the label of its only neighbor. Then eliminate the leaf from the tree and repeat the process. This sequence of labels forms the Prüfer coding of the tree. If we count the number of occurrences of a number in this sequence, it equals the degree of its vertex minus 1.