Polya Conjecture

n

20000

view

odd kind minus n/2

odd and even kinds

Define a number as of odd kind if the number of its prime factors is odd (taking multiplicity into account), and to be of even kind if the number of prime factors is even. Let

E(n)

and

O(n)

be the sum of the numbers of integers less than or equal to

n

of the even and odd kinds.

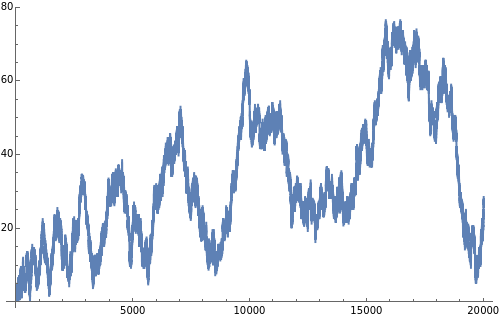
Polya conjectured in 1919 that for all

n>2

,

O(n)≥E(n)

. In 1962, Lehman found the first counterexample: 906150257. The first plot is of

O(n)-n/2

, which is closely related to the Liouville function

λ

. It shows fluctuations with increasing maxima alternating with minima near the

n

axis. The second plot shows

O(n)

versus

E(n)

.