Perturbing the Constant Coefficient of a Complex Polynomial

m

1

2

3

4

5

6

7

m

2

1

2

3

4

m

3

1

2

3

z 1
z 2
z 3
a

Axes

plot range

0.5

1

2

3

4

plot points factor

1

2

3

4

5

6

2

(z-1)

5

z

3

(z+0.5)

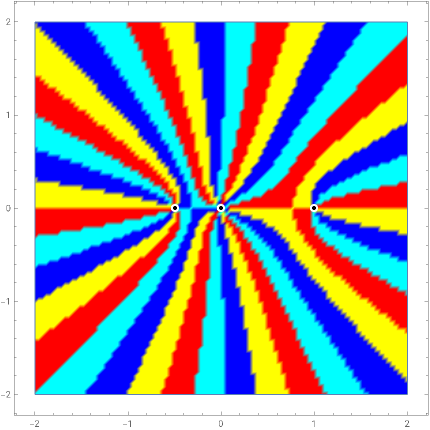
This Demonstration plots the complex polynomial

P(z)=

m

1

(z-

z

1

)

m

2

(z-

z

2

)

m

3

(z-

z

3

)

+a

. For

a=0

, the multiplicity of a root determines the number of cycles (cyan, blue, yellow, red) around the root (shown as a black dot). An example is shown in the first snapshot. You can vary

z

1

,

z

2

,

z

3

,

m

1

,

m

2

,

m

3

and

a

. Small changes in the constant term, which makes

a≠0

, transforms the polynomial to a form

∏

k

(z-

z

k

)

, with all of the roots of the new polynomial being simple.