Orbit of a Launched Satellite

mass of planet

6.×

24

10

initial altitude

x

1

600000

launch speed

v

1

19000

planet mass = 6.×

24

10

kg

x

2

= 222.61 km

v

2

= 51210.5 m/s

eccentricity = 0.458771

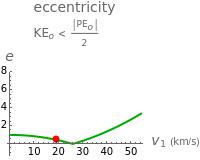
Kepler observed that the path of a satellite in an orbit around a heavy mass traces out an ellipse. More generally, an orbit in the absence of a third body is always a conic section (i.e., an ellipse, parabola, or hyperbola). For a parabolic orbit, the eccentricity equals 1, for any value of the initial launch speed. This speed along with the planet's mass, the initial altitude and the gravitational constant,

G

, determine the eccentricity of the conic section traced by the satellite's orbit. If the orbit is closed, then the speed is always greatest at the smallest distance from the planet and smallest at the greatest distance from the planet. This is derived from conservation of angular momentum.

An image of a double cone and its intersection with a plane traces out the corresponding conic for the satellite's trajectory.