Nowhere-Neat Tilings of the Plane
Nowhere-Neat Tilings of the Plane
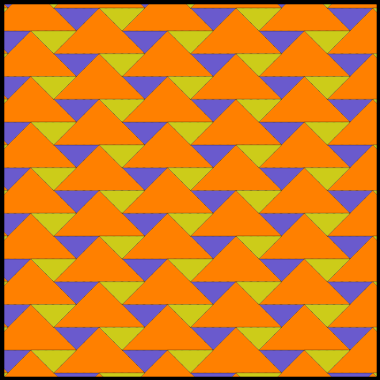
If no two tiles in a (polygonal) tiling have a full side in common, the tiling is called nowhere-neat.
Related mathematical problems: to find nowhere-neat tilings of -gons with -gons and to find nowhere-neat tilings of the plane with only a few prototiles.
n
m
The Demonstrations "Nowhere-Neat Tilings" and "Nowhere-Neat Squaring the Square" by the same author were dedicated to the first of these two problems.
This Demonstration deals with the latter case of covering the infinite plane in a nowhere-neat way while using only one or two prototiles. In fact, it only deals with tilings on the integral grid.
There are over 200 tilings shown in this Demonstration; maybe you can find a few more.