Gregory Series

n

4

π

4

≈

4

∑

k=1

k+1

(-1)

2k-1

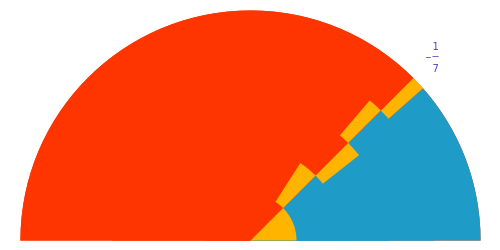
The Gregory series,

1-

1

3

+

1

5

-

1

7

+…

, is a slowly converging formula for

π

4

found in the 1670s by James Gregory and Gottfried Wilhelm Leibniz, each working independently. It is obtained by substituting

x=1

into the Leibniz series

arctan(x)=

∞

∑

k=1

k+1

(-1)

k+1

x

2k-1

. The number near

π

4

on the circle is the last term added.