Feynman's Relativistic Electrodynamics Paradox

v

1

0.

v

2

0.

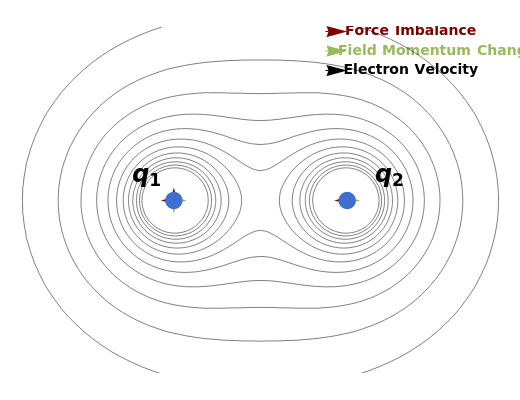
A charged particle is located on the



x

axis with its velocity pointing along the



x

axis. Another charged particle is also located on the



x

axis, but with its velocity pointing along the negative



y

axis. The net relativistic electric force on both particles is given by

Δ

F

E

=

q

2



E

(

x

2

)+

q

1



E

(

x

1

)=

1

2

γ

1

-

γ

2

k

q

1

q

2

(

x

2

-

x

1

)



x

, where

k

is the Coulomb constant,

γ

i

is the factor

1

1-

2

ν

1



2

c

,

q

i

is the charge, and

χ

i

is the position of the

th

i

particle. The relativistic magnetic force of particle 2 on 1 is

F

B

=

ν

1

ν

2

k

q

2

q

1

γ

2

c

2

(

x

2

-

x

1

)



y

. The magnetic force of particle 1 on 2 is zero. The momentum of the electromagnetic field is given by the time derivative of the vector potential

A

C

in the Coulomb gauge,

q

d

A

C

dt

=q

∂

A

C

(t)

∂t

|

t=0

=

k

q

1

q

2

ν

1

2

c

(

x

2

-

x

1

)

+

k

q

2

q

1

2

(

x

2

-

x

1

)

(

γ

2

-1)



x

+

-k

q

2

q

1

ν

2

ν

1

γ

2

c

(

x

2

-

x

1

)



y

. The sum of all three terms is zero and shows momentum conservation. The contour lines show the relativistic Liénard–Wiechert potential for each charge.