Equilibrium of a Rigid Bar

weight of block

51

placement of block

2.9

F

C

= (

d

2

-

d

1

) / (

d

3

-

d

1

)

F

A

= 3.90 / 10. × 51 = 19.89

F

B

=

F

A

-

F

C

= 51 - 19.89 = 31.11

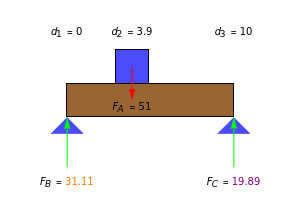
A rigid bar of negligible mass is supported on either end by two triangular blocks. On top of the bar sits a box of variable mass that can be moved along the bar. The forces

F

B

,

F

A

, and

F

C

act at

d

1

,

d

2

, and

d

3

.

This Demonstration shows how to use balancing of force and torque to find the forces exerted by the triangular blocks on the bar due to the weight of the box.

The sum of the forces in the

x

or

y

direction,

F

x

and

F

y

, must be zero. In the

y

direction this means that the force exerted by the block on the bar,

F

A

, must be balanced by the forces from the triangular blocks on the bar,

F

B

and

F

C

; therefore

F

A

=

F

B

+

F

C

, or

F

B

=

F

A

-

F

C

. There are no forces acting in the

x

direction.

The sum of the counterclockwise torques must equal the sum of the clockwise torques. In this case this means that

(

d

2

-

d

1

)

F

A

=(

d

3

-

d

1

)

F

C

, or

F

C

=(

d

2

-

d

1

)/(

d

3

-

d

1

)

F

A

.