Difference Formula for Cosine

angle A

angle B

The difference formula for cosine states that

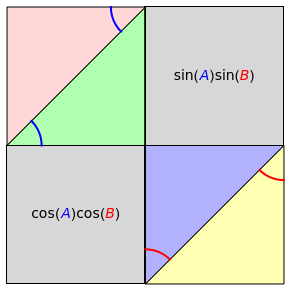
cos(A-B)=cos(A)cos(B)+sin(A)sin(B)

.

All triangle hypotenuses in the above figures are of unit length, so that the sines and cosines are simply the adjacent or opposite sides of their triangles relative to the angles

A

,

B

, or

A+B

. The gray areas on the left and right equal the left and right sides of the formula. The angle at the black dot on the left is equal to

π/2-(A-B)

, so the dashed line (and thus the gray area) is the sine of this angle, which is equal to

cos(A-B)

by one of the cofunction identities.

When

A=B

, setting

x=2A

, the formula reduces to

1=

2

cos

(x)+

2

sin

(x)

, which is essentially Pythagoras' theorem.

When

B=-A

, the formula reduces to the double-angle formula for cosine,

cos(2A)=

2

cos

(A)-

2

sin

(A)

.