Convergence of the Empirical Distribution Function of a Sample
Convergence of the Empirical Distribution Function of a Sample
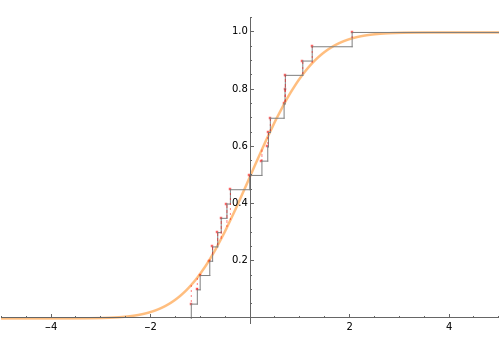
The empirical distribution function (EDF) of a random sample is the cumulative distribution function of the values obtained in the sample. You would intuitively expect the EDF to resemble the cumulative distribution function of the parent distribution (that is, the distribution the sample is drawn from).
This idea is formalized in the Glivenko-Cantelli theorem (also called the fundamental theorem of statistics), which states that the EDF converges pointwise to the parent distribution.
This result is illustrated here for three different parent distributions: the normal distribution with parameters (0, 1), the uniform distribution with parameters (-5, 5), and the gamma distribution with parameters (2, 0.6). You can see the EDF of a random sample approach the parent distribution as the sample size increases.