Complex Spherical Harmonics
Complex Spherical Harmonics
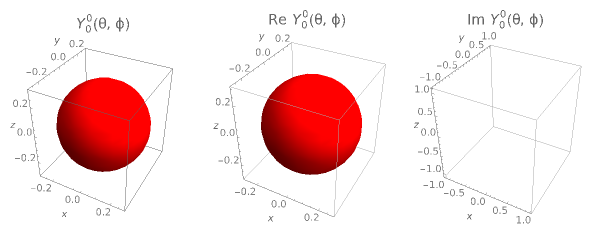
Spherical harmonic functions arise for central force problems in quantum mechanics as the angular part of the Schrödinger equation in spherical polar coordinates. They are given by (θ,ϕ)=(cosθ), where are associated Legendre polynomials and and are the orbital and magnetic quantum numbers, respectively. The allowed values of the quantum numbers, which follow from the boundary conditions of the problem, are . The complex function (θ,ϕ) is shown on the left, where the shape is its modulus and the coloring corresponds to its argument, the range 0 to corresponding to colors from red to magenta. The center and right graphics show the corresponding real and imaginary parts.
m
Y
ℓ
(2ℓ+l)
4π
(ℓ-|m|)!
(ℓ+|m|)!
m
P
ℓ
mϕ
e
m
P
ℓ
l
m
ℓ=0,1,2,...,∞,m=0,±1,±2,...,±ℓ
m
Y
ℓ
2π