You are using a browser not supported by the Wolfram Cloud
Supported browsers include recent versions of Chrome, Edge, Firefox and Safari.
I understand and wish to continue anyway »
WOLFRAM NOTEBOOK
A Path through the Lattice Points in a Quadrant
A Path through the Lattice Points in a Quadrant
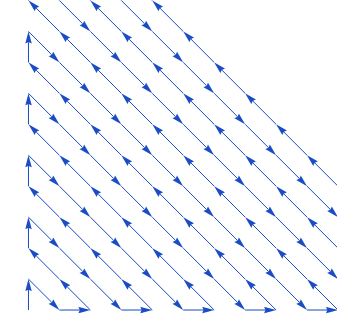
Let ={1,2,3,…} be the set of positive integers. The set of lattice points in the first quadrant is the set ×={(1,1),(1,2),…}, where both coordinates are positive integers. Even though × is two-dimensional, it is possible to set up a one-to-one correspondence between × and , as shown in the picture.
+
+
+
+
+
+
+
+
By associating with the lattice point , the path through the lattice points gives an enumeration of the positive unreduced rational numbers. Skipping past the fractions that have a common factor gives a listing of the positive rational numbers. The matching shows that there are as many positive fractions as positive integers.
m
n
(m,n)
In spite of that, there are differences between the integers and the rationals; for example, between any two rationals there is another rational.