You are using a browser not supported by the Wolfram Cloud
Supported browsers include recent versions of Chrome, Edge, Firefox and Safari.
I understand and wish to continue anyway »
WOLFRAM NOTEBOOK
A Concurrency Generated by Circles about a Triangle's Sides and Lines through an Internal Point
A Concurrency Generated by Circles about a Triangle's Sides and Lines through an Internal Point
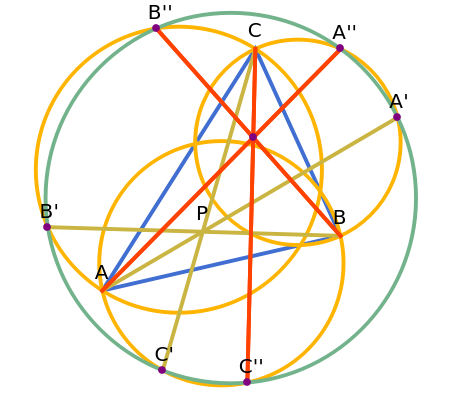
Let ABC be a triangle and P be an interior point. Let A', B', and C' be the external intersections of AP, BP, and CP with the circles whose diameters are BC, AC, and AB, respectively. Let the circumcircle of A', B', and C' intersect the circles whose diameters are BC, AC, and AB again at A'', B'', and C''. Then AA'', BB'', and CC'' are concurrent.