4. Constructing a Point on a Cassini Oval

lengths of major and minor axes

a

0.87

b

1.53

point

D

1

on hyperbola

1.19

another point D on hyperbola

-0.3

show Cassini oval

|

D

1

E

1

||

D

2

E

2

|=9.23153

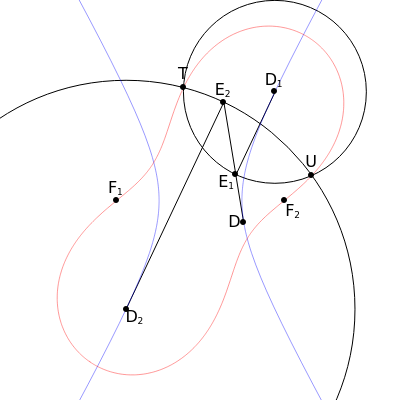
This Demonstration shows another construction of Cassini's oval. Start with the hyperbola

H

with equation

2

x

2

a

-

2

y

2

b

=1

of eccentricity

ϵ=

2

a

+

2

b

a

,

a,b>0

. Select any point

D

1

(u,v)

on

H

. Let

D

2

be the opposite point of

D

1

and

D

a point on

H

different from

D

1

and

D

2

. The tangents on

H

at

D

1

and

D

2

are parallel and meet the tangent at

D

at points

E

1

and

E

2

, respectively. Then

D

1

E

1



D

2

E

2

=-

2

a

+

2

ϵ

2

b

.

Draw a circle with center

D

1

and radius



D

1

E

1



and a circle with center

D

2

and radius



D

2

E

2



; suppose these meet in points

T

and

U

. But then



D

1

T

D

2

T=-

2

a

+

2

ϵ

2

b

. So

T

is a point on a Cassini oval with foci

D

1

and

D

2

. The same is true for the point

U

. It can be shown that the foci

F

1

and

F

2

are also on the oval.